Mathematics is a fascinating world where patterns and properties govern the relationships between numbers. One of the fundamental concepts in this realm is the commutative property. Whether you’re a student trying to grasp basic math concepts or an adult looking to brush up on your knowledge, understanding the can enrich your comprehension of mathematical operations. Let’s explore this property in depth.
What is the Commutative Property?

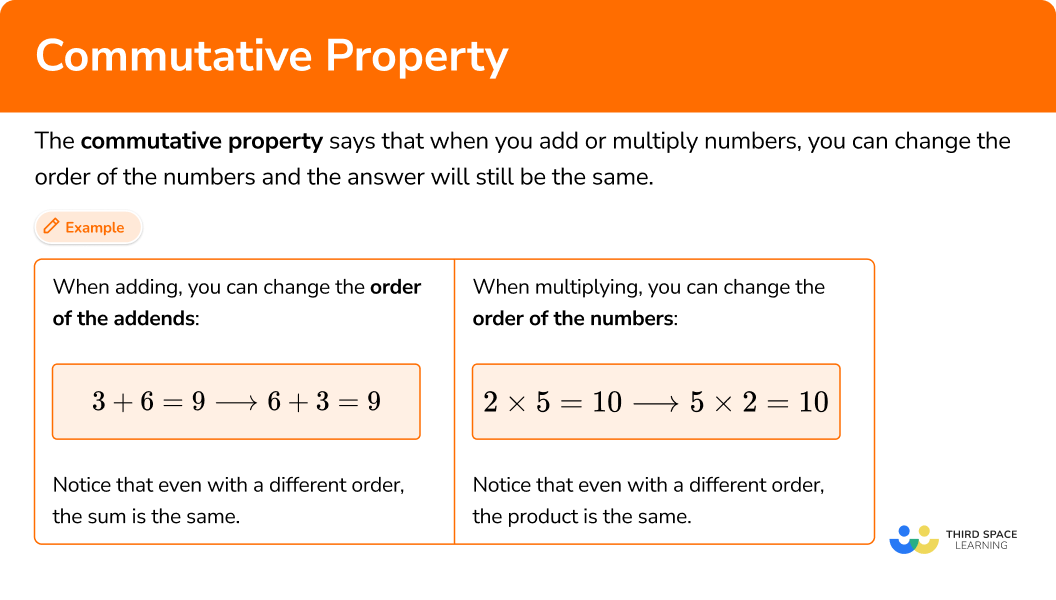
The commutative property is a foundational principle in mathematics that states that the order in which two numbers are added or multiplied does not affect the outcome. In simpler terms, if you have two numbers, say aaa and bbb, the tells us that:
- For addition: a+b=b+aa + b = b + aa+b=b+a
- For multiplication: a×b=b×aa \times b = b \times aa×b=b×a
This property is not just a quirk of math; it’s a crucial building block that supports a vast array of mathematical concepts and operations.
A Closer Look at Addition
Let’s take a closer look at addiction and how the commutative property plays a role. Imagine you’re in a café, and you’re trying to calculate the total cost of your drinks. If you ordered a coffee for $3 and a pastry for $2, you can add them in either order:
- 3+2=53 + 2 = 53+2=5
- 2+3=52 + 3 = 52+3=5
No matter how you slice it, the total remains $5. This is the essence of the in action. It’s a handy feature that simplifies calculations and helps us to think flexibly about numbers.
Multiplication Made Easy
The commutative property also extends to multiplication. Let’s stick with the café example. If you decide to buy two coffees and three pastries, you can calculate the total in different ways:
- 2×3=62 \times 3 = 62×3=6
- 3×2=63 \times 2 = 63×2=6
Again, the total remains the same. This flexibility is especially helpful in more complex calculations where rearranging numbers can make the math easier to manage.
Why is the Commutative Property Important?
Understanding the commutative property is crucial for several reasons. First, it enhances numerical fluency, making it easier for individuals to perform mental calculations. When you know you can rearrange numbers, you can often find simpler ways to solve problems.
Building Blocks for Advanced Math
The commutative property lays the groundwork for more advanced mathematical concepts. For example, when dealing with algebraic expressions, recognizing that you can rearrange terms allows you to simplify expressions more efficiently. This is particularly valuable in higher mathematics, where complex equations are the norm.
Enhancing Problem-Solving Skills
Moreover, embracing the cultivates better problem-solving skills. When faced with a complex equation, being able to rearrange components can open up new pathways to solutions that might not be immediately obvious. This flexibility is invaluable in mathematics and related fields, like engineering and computer science.
Commutative Property in Everyday Life
You might be surprised to learn that the commutative property isn’t just a dry mathematical principle; it shows up in our everyday lives more often than we realize.
Shopping and Budgeting
Consider budgeting. When you list your expenses, it doesn’t matter in which order you add them up. If you spend $20 on groceries and $15 on a dinner out, your total remains consistent no matter how you calculate it:
- $20 + $15 = $35
- $15 + $20 = $35
This consistency is reassuring when you’re managing finances, as it allows for flexible thinking about your spending.
Cooking and Baking
Cooking also utilizes the commutative property. Suppose you’re making a fruit salad with apples, bananas, and oranges. It doesn’t matter in which order you add the fruits to the bowl; the final product will be the same delicious salad.
Sports Statistics
In sports, the commutative property can come into play when calculating points or scores. For example, if a player scores 5 points in the first quarter and 3 in the second, it doesn’t matter how you add those points; the total remains the same, providing a clear picture of their performance.
Limitations of the Commutative Property
While the commutative property is widely applicable, it does have its limitations. It’s essential to recognize where this property applies and where it doesn’t.
Non-Commutative Operations
The commutative property does not apply to all mathematical operations. For instance, subtraction and division are non-commutative. If you have a=5a = 5a=5 and b=3b = 3b=3:
- 5−3≠3−55 – 3 \neq 3 – 55−3=3−5 (the results are different)
- 5÷3≠3÷55 \div 3 \neq 3 \div 55÷3=3÷5
Understanding these exceptions is crucial for accurate calculations and reinforces the need for careful consideration when performing operations.
Complex Numbers
In more advanced mathematics, such as with matrices or certain algebraic structures, the commutative property may not hold. For example, matrix multiplication is generally non-commutative, meaning that the order in which you multiply matrices matters significantly.
Real-World Applications
Recognizing the limitations of the commutative property can also enhance critical thinking and problem-solving skills. In fields like physics or engineering, knowing when the does not apply can prevent errors in calculations that could have serious implications.
Teaching the Commutative Property
For educators, conveying the concept of the commutative property can be both fun and engaging.
Interactive Activities
One effective way to teach this concept is through interactive activities. For example, using physical objects like blocks or counters can help students visualize the idea. By physically rearranging objects, students can see that the total remains constant regardless of how they group or arrange them.
Games and Challenges
Incorporating games can also make learning the commutative property enjoyable. Math puzzles that require students to rearrange numbers to achieve the same sum or product can playfully reinforce the concept.
Real-World Contexts
Using real-world contexts, like shopping scenarios or cooking, can help students relate to the commutative property. When students see the relevance of math in their daily lives, they are more likely to engage with and understand the material.
The Commutative Property in Algebra
As students progress in their mathematical education, the commutative property becomes increasingly important in algebra.
Simplifying Expressions
In algebra, students often encounter expressions that require simplification. The ability to rearrange terms, such as 3x+5x3x + 5x3x+5x to 5x+3x5x + 3x5x+3x, leverages the commutative property to streamline calculations. This skill is vital as students tackle more complex equations.
Solving Equations
When solving equations, recognizing that you can rearrange terms can lead to quicker solutions. For instance, if you have an equation like 2x+3=5+x2x + 3 = 5 + x2x+3=5+x, knowing you can rearrange and combine as terms will aid in finding the value of xxx.
Graphing and Functions
The commutative property also plays a role in graphing functions. When plotting points, the order in which you label the axes or the sequence of points plotted doesn’t change the overall representation. This property is particularly useful when visualizing algebraic concepts.
Commutative Property vs. Associative Property
While the commutative property is a key mathematical concept, it is essential to differentiate it from the associative property.
Defining the Associative Property
The associative property deals with grouping rather than order. For addition and multiplication, the associative property states:
- For addition: (a+b)+c=a+(b+c)(a + b) + c = a + (b + c)(a+b)+c=a+(b+c)
- For multiplication: (a×b)×c=a×(b×c)(a \times b) \times c = a \times (b \times c)(a×b)×c=a×(b×c)
Comparing the Two Properties
While both properties allow for flexibility in calculations, they serve different purposes. The commutative property focuses on the order of numbers, whereas the associative property emphasizes how numbers are grouped. Understanding these distinctions is crucial for mastering more complex mathematical concepts.
Practical Implications
In practical terms, knowing both properties can simplify calculations. For example, when calculating the total cost of multiple items, you can rearrange or regroup your numbers to make the math easier, demonstrating the effectiveness of both the commutative and associative properties in action.
Real-Life Examples of the Commutative Property
Real-life scenarios provide excellent illustrations of the commutative property.
Planning a Trip
When planning a trip, you might consider various travel routes and stops. Whether you visit the museum before the park or vice versa, your overall experience doesn’t change based on the order of the visits.
Team Sports
In team sports, the commutative property can be observed in scoring. A basketball team can score points in any order throughout the game. The total score at the end remains the same regardless of who scores first or how many points they contribute.
Cooking and Recipes
In cooking, you can add ingredients in any order. Whether you mix flour and sugar before adding eggs or the other way around, the final batter will remain the same, illustrating the practical implications of the commutative property.
Conclusion: Embracing the Commutative Property
The commutative property is a cornerstone of mathematical understanding, offering flexibility and simplicity in calculations. Recognizing its applications and limitations enhances our problem-solving skills and fosters a deeper appreciation for the beauty of mathematics. Whether you’re teaching this concept, applying it in real-life scenarios, or exploring more advanced mathematics, the commutative property remains an essential tool in your mathematical toolkit. Embrace it, and you’ll find that math becomes a more accessible and enjoyable journey!